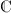

where 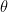 is the longitude and
is the longitude and  the latitude. R is the radius of the sphere, which is 1 in our program.
the latitude. R is the radius of the sphere, which is 1 in our program. 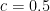 and
and  are two constants controlling the shape of the projection.
are two constants controlling the shape of the projection.
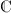

where 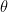 is the longitude and
is the longitude and  the latitude. R is the radius of the sphere, which is 1 in our program.
the latitude. R is the radius of the sphere, which is 1 in our program. 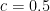 and
and  are two constants controlling the shape of the projection.
are two constants controlling the shape of the projection.
StatsPage (last edited 2011-07-07 19:11:38 by WikiAdministrator)